Indexed In
- Open J Gate
- JournalTOCs
- RefSeek
- Hamdard University
- EBSCO A-Z
- OCLC- WorldCat
- Scholarsteer
- Publons
- Geneva Foundation for Medical Education and Research
- Google Scholar
Useful Links
Share This Page
Journal Flyer

Open Access Journals
- Agri and Aquaculture
- Biochemistry
- Bioinformatics & Systems Biology
- Business & Management
- Chemistry
- Clinical Sciences
- Engineering
- Food & Nutrition
- General Science
- Genetics & Molecular Biology
- Immunology & Microbiology
- Medical Sciences
- Neuroscience & Psychology
- Nursing & Health Care
- Pharmaceutical Sciences
Research Article - (2021) Volume 9, Issue 9
Development of regression model to investigate the correlation of selected input parameters to the thread consumption of over-lock stitch 514
T.S. Niroshan*Received: 09-Dec-2020 Published: 24-Sep-2021, DOI: 10.35248/2329-6798.21.9.321
Abstract
The cost of manufacturing and distribution of apparel products are highly depended on the sewing thread consumption. Thus, the calculation of sewing thread consumption has to be done accurately using effective methods. Due to the inefficiency of the existing formulae, the predictions of thread requirement became inaccurate. The consumption calculations exhibit significant error percentages due to the ignorance of important parameters which effect on thread consumption. This study investigates on correlation of many parameters such as GSM, seam width, SPI, frequency, thickness of fabric and yarn count to thread consumption of over-lock stitch 514. The existing thread consumption formulae are optimized by considering new parameters, using regression analysis and geometrical modelling techniques. For the over-lock stitch 514, results indicate that above parameters significantly affect in determination of the thread consumption. This particular method addresses the following issues: In accurate consumption calculation, Excess seam length or inaccurate seam length, Use of predefined thread consumption factors and use to calculate thread consumption by only considering machine type. The error analysis of proposed formulae was performed to indicate that the proposed formulae more accurate compared to the current method of predicting sewing thread consumption. Therefore, the proposed formulae are expected to be a better approach to calculate thread consumption of over lock stitch 514.
Keywords
GSM, Over-lock Stitch, Thread consumption, Seam Length, Regression Model
Introduction
Sewing thread is considered as strategic supply for garment industry among the consumed important quantities[1]. Cost is an important consideration in selecting thread after its performance and appearance in terms of aesthetics. Total thread costs constitute the costs of the thread used in a production run of garments, the thread wasted during sewing, and the thread stock remains unused at the end of a contract. Apart from that it includes the cost that can arise in production or during the subsequent use of the garment because the thread was faulty[2]. It is obvious that the precise defining of required sewing thread will reduce the unused stocks in the industry as the required amount will be available for production.
The major goal of this study is accurate determination of the required quantity of thread and the estimation of corresponding actual costs. Thread consumption can vary within the garments of same type as well. Differences in size, style, and material of the garment determine the amount of thread used[3]. Thread consumption is also directly related to the factors namely stitch length, stitch density, thickness of material and width of the seam, number of plies, thread type and tension of the thread[4]. This particular study establishes an inter-relationship among the selected parameters through the regression model.
Methodology
Experiment was designed to evaluate the variation of thread consumption with respect to the considered parameters: GSM, number of plies, seam width, stitch density (SPI), thickness of the fabric, yarn count and tension of the thread. The experiment was conducted using single jersey, slub jersey and fleece fabrics.
As per the stitch selected; 4 thread over lock (514), recommended thread types of cotton core-spun thread and polyester continuous filament yarn thread were used. Yarn count of the thread was taken as the thread ticket number value. Fabric thickness was measured by thickness measuring gauge. Thread consumption was measured by manually by removing the thread carefully from the seam, measured by grading ruler manually and average thread tension were measured using thread tension measuring gauge with the accuracy up 0.1 cn. All the tension values were measured at the positions closer the needle. GSM was measured by cutting plate and the electric balance.
Experiment was designed by selecting different value ranges of concerned parameters. The value ranges of GSM, number of plies, seam width, stitch density, yarn count, tension and fabric thickness were selected in a manner that as they enable a balance stitch in all the sewn specimen.
Below table includes these input parameters and the set up values for each experiment. Each stitch was sewn some inch of lengths and then cut 1, 2 or 3 inches from each specimen to measure the thread lengths. In 4 threads over lock seam there are 2 needle threads and 2 needle looper threads.
| Variables | Value used |
|---|---|
| GSM | 24,22,59,26,52,79,308 |
| Number of plies | 1,2,3,5 |
| Seam width (mm) | 5,6,7,8 |
| SPI | 13,14,15,16 |
| Thickness of Material(mm) | 0.61,0.69,0.7,0.74,0.78 |
| Thread count (Tex) | 12,01,60,180 |
| Thread tension (cN) | From 40 to270 |
Table 1: Input Parameters
Unravelling thread was a difficult task without stretching the threads.
According to the data collections the equations are created by excel and automatically the consumption of thread can easily calculated by it correctly.
Results and Discussion
The influence of fabric weight
A change of fabric weight has a great effect on the thread consumption. The mass or the weight (g/mm2) has a little influence on the thread consumption of sewing garments. From the estimation of consumption in heavy fabrics, it is obvious that they consume more sewing thread than an average fabric[5].
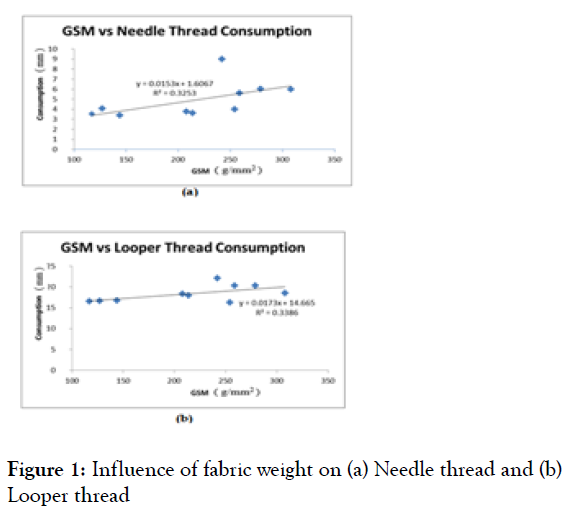
Figure 1: Influence of fabric weight on (a) Needle thread and (b) Looper thread
The Influence of number of plies in the seam
Seam thickness is directly influences the thread consumption. Because through the fabric the needle thread has to travel and looper thread also should cover all the seam thickness [6]. If the seam thickness is high the consumption of thread is also high. If the number of plies increases then the seam thickness also increases.
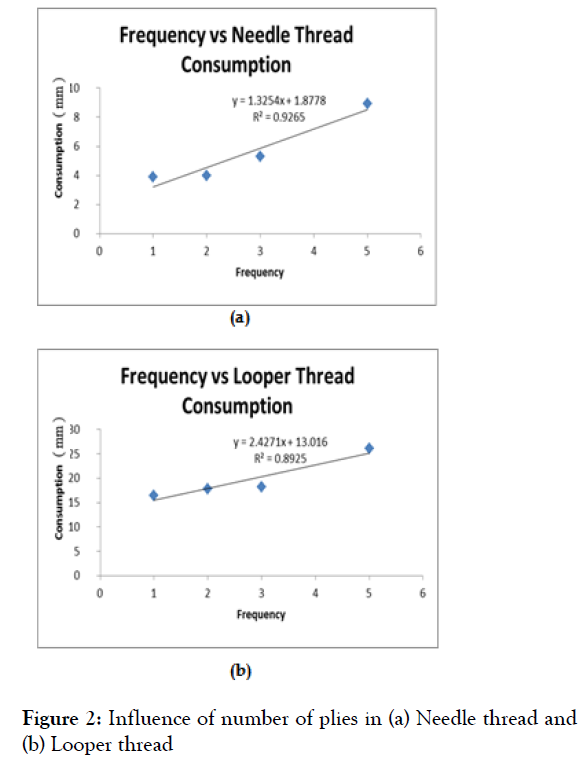
Figure 2: Influence of number of plies in (a) Needle thread and (b) Looper thread
Influence of width of the seam
Normally in 4 thread over-lock stitches using 1/8 inches or 1/4 inches space between needles. So that with increasing the seam width the consumption of thread is also increased. According to the SPI and tension of the thread the seam width can be vary. There are other parameters such as thread type and fabric type influence the seam width indirectly.
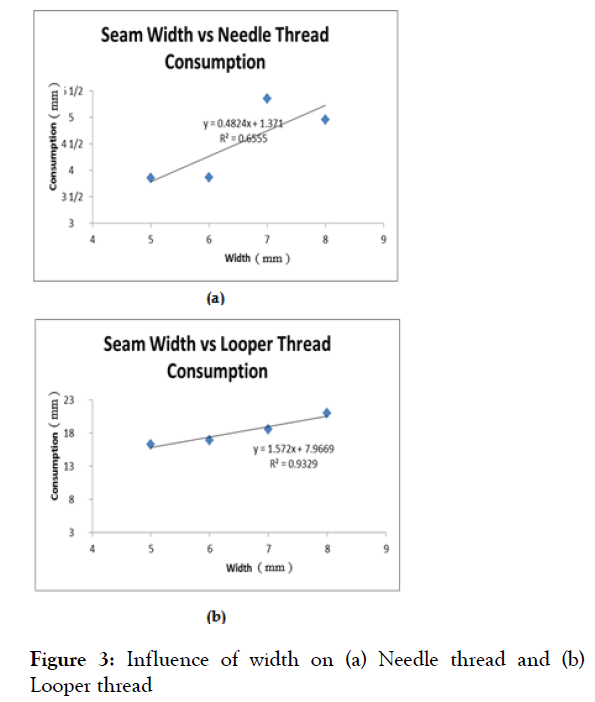
Figure 3: Influence of width on (a) Needle thread and (b) Looper thread
The influence of stitches per inch (SPI)
Stich length which is defined as the number of stitches, has a remarkable influence on the variation of sewing thread consumption[8]. Moreover, the results show that higher the length of the stitch, higher is the consumption of thread. Indeed this is due to the increase in number of bent thicknesses and of the formed lockstitch number. It confirms the finding that with the fabric feed in motion the thread is required to form the upper and lower lengths during stitch length modification.
The upward excursion of the take-up Lever due to its approach to the top of its vertical travel during the fabric progression creates a demand for thread. Consequently, the thread consumption modified as the needle-thread which is required to form increasingly large loop to form the stitch length affects the geometric profile of the lockstitch. The increase of the number of stitches per inch means the decrease of stitch length geometry which allows a reduction in needle thread consumption as shown below. It proves that if the sewing length decreases then thread consumption also decreases.
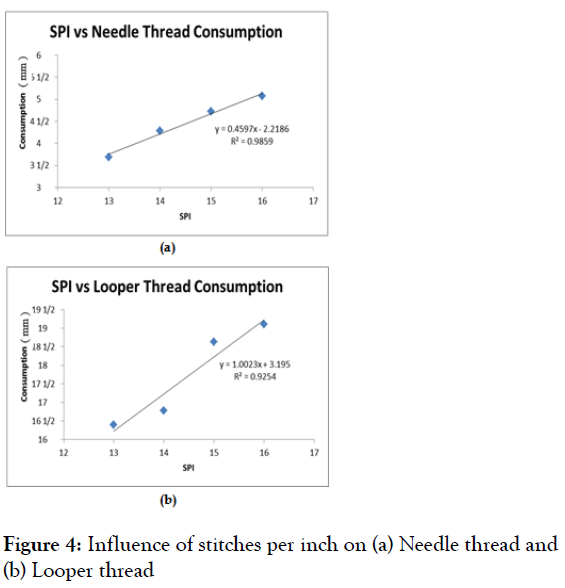
Figure 4: Influence of stitches per inch on (a) Needle thread and (b) Looper thread
The influence of thickness of fabric
The needle is penetrated through the fabric and needle thread consumption is directly depends on thickness of fabric, same as the number of plies influences the thread consumption the looper thread is increased while the thickness of the seam is increased. So if the thickness of the fabric increases obviously the seam thickness is also increased. The below graphs are clearly shown that the thread consumption is directly influenced on consumption of thread.
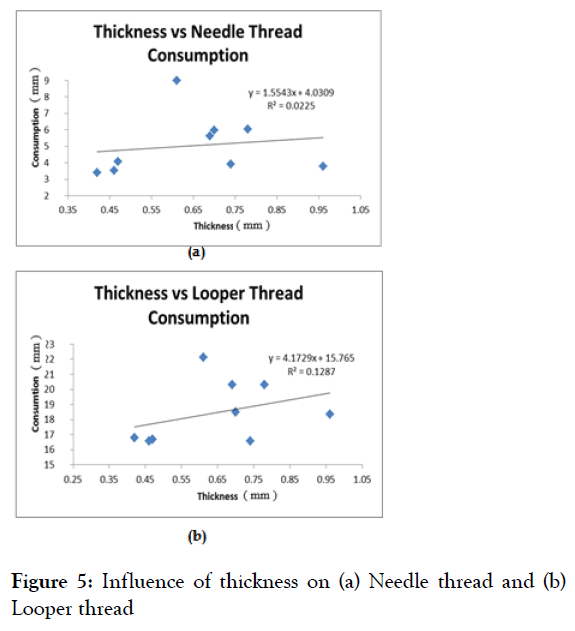
Figure 5: Influence of thickness on (a) Needle thread and (b) Looper thread
The influence of threat count
Ticket number is used to represent the thread count in the industry. With the increasing of ticket number the thickness of the threads is reduced. It’s also an impact in thread consumption in needle and looper as shown in the below graphs.
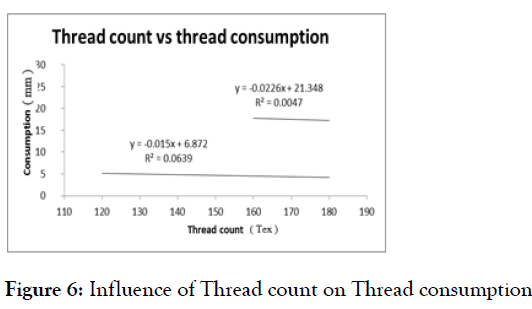
Figure 6: Influence of Thread count on Thread consumption
The influence of threat tension
According to the tension of thread can cause the variance in thread consumption because there are two needle threads and two looper threads which are having different tensions individually. But based on the actual data these below graphs are showing that there are some variations in thread consumption while tension of threads is changing.
Figure 7: Influence of Thread tension on thread consumption
From the results of multiple linear regression analysis, it is displayed that the effect from tension of the needle thread as a combinatorial effect of tension together with another input variable is less significant. Since the tension is not a big impact on the thread consumption theoretically the tension can be omitted from the equation [7].
The derived formula for four thread over-lock stitch 514 is denoted for needle thread in
514Needle= 0.0221161834*M + 1.344711569*f -0.1746*w + 0.320603*s - 0.870141*t + 0.02762*T1 -0.06157*T2 - 1.00814 … (1)
The derived formula for four thread over-lock stitch 514 is denoted for looper thread in
514Looper = -0.019874037*M + 1.5103595*f + 1.865103*w + 0.913904*s + 7.841488*t -0.0576079*T1 + 0.091804*T2 - 17.6912 …(2)
Where,
M- Grams per square metre (GSM)
f- Number of Plies
w- Seam width in mm
s- Stitches per inch (SPI)
t- Thickness of material in mm
T1- Needle thread count in Tex
T2- Looper thread count in Tex
N1, N2- Needle thread
L1, L2- Looper thread

Figure 8: Stitch type 514
Experimentation
Experiment 01
| Black Spun | Black Yarn | |
|---|---|---|
| Ordered thread cones | 57 | 154 |
| Experimental results | 68*1.03=70mm | 111*1.03=115mm |
| Actual consumption | 70mm | 117mm |
Table 2: Experiment 01
The actual consumption and experimental results are approximately equal
Experiment 02
| Pure black Spun | Pure black Yarn | |
|---|---|---|
| Ordered thread cones | 93 | 278 |
| Experimental results | 99*1.03=102mm | 271*1.03=279mm |
| Actual consumption | 112mm | 276mm |
Table 3: Experiment 02
When considering all the parameters which are influenced in the thread consumption while making the prediction for thread amount for a garment, it will be approximately satisfied the actual thread consumption.
Therefore during the production there will be no shortage for thread cones as well as less number of excess cones. So it can be eliminated in the production difficulties and cost by considering all the parameters which are affecting the actual thread consumption.
Conclusion
The proposed investigation is limited only for four threads over lock stitch and usage only one is not adequate to apply it in apparel business environment where several types of stitches are used. The investigation need to be extended for all 6 classes of stitches so that industry will be able to use deriving formula in an effective way[8]. This particular formulae will work under the defined value ranges of corresponding parameters and there are possibilities for further analysis as well. Investigation of learning with more parameters such as machine tension, fabric tension, and thread elongation will be the suggesting areas to explore as future methods of sewing thread consumption predictions.
The amount of sewing thread consumed can be calculated by keeping few parameters constant. The unchanged parameters are sewing thread type, SPI and seam width. Also the programme can calculate the consumed sewing thread amount with only 3, 4 and 5 stitch densities and only for four-thread over lock stitch.4.
REFERENCES
- M. Jaouadi, S. Msahli, A. Babay, and B. Zitouni, “Analysis of the modeling methodologies for predicting the sewing thread consumption,” Int. J. Cloth. Sci. Technol., Jan. 2006.
- A. Rasheed, S. Ahmad, M. Mohsin, F. Ahmad, and A. Afzal, “Geometrical model to calculate the consumption of sewing thread for 301 lockstitch,” J. Text. Inst., (2014), 105: 1259–1264.
- Vi. K. Midha, S. Sharma, and V. Gupta, “Predicting sewing thread consumption for lockstitch using regression model,” Res. J. Text. Appar., (2016).
- J. Fan and W. Leeuwner, “The Performance of Sewing Threads with Respect to Seam Appearance,” J. Text. Inst., (1998), 89: 142–154.
- M. Sarah, J. Boubaker, K. Faouzi, and D. Adolphe, “Sewing Thread Consumption for Different Lockstitches of Class 300 Using Geometrical and Multi-Linear Regression Models,” Autex Res. J., (2019), 1.
- R. P. Abeysooriya and G. L. D. Wickramasinghe, “Regression model to predict thread consumption incorporating thread- tension constraint: study on lock-stitch 301 and chain-stitch 401,” Fash. Text., (2014), 1: 14
- S. Mandal, “Studies on seam quality with sewing thread size, stitch density and fabric properties,” Thesis, The Hong Kong Polytechnic University, 2009.
- J. Boubaker, F. Khedher, and F. Mili, “Consumption of the sewing thread of jean pant using Taguchi design analysis,” Autex Res. J., (2012), 12.
Citation: Niroshan TS (2021) Development of regression model to investigate the correlation of selected input parameters to the thread consumption of over-lock stitch 514. Mod Chem Appl. 9:p111
Copyright: © 2021 Niroshan TS. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.